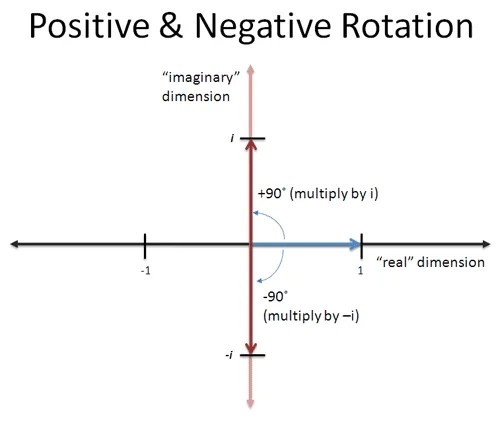
This article is related, and a little similar, to another recent article. While researching, I was simultaneously reading through both a Wikipedia entry and a Better Explained discussion on a subject I know fairly well (Imaginary Numbers). The articles were saying the same things from different directions, and when I looked at a certain illustration, suddenly I could see more in it than I ever had before. Look closely at these two images, you may be able to spot the key difference also:
 |
 |
| first image from Better Explained, 2nd image is from Wikipedia | |
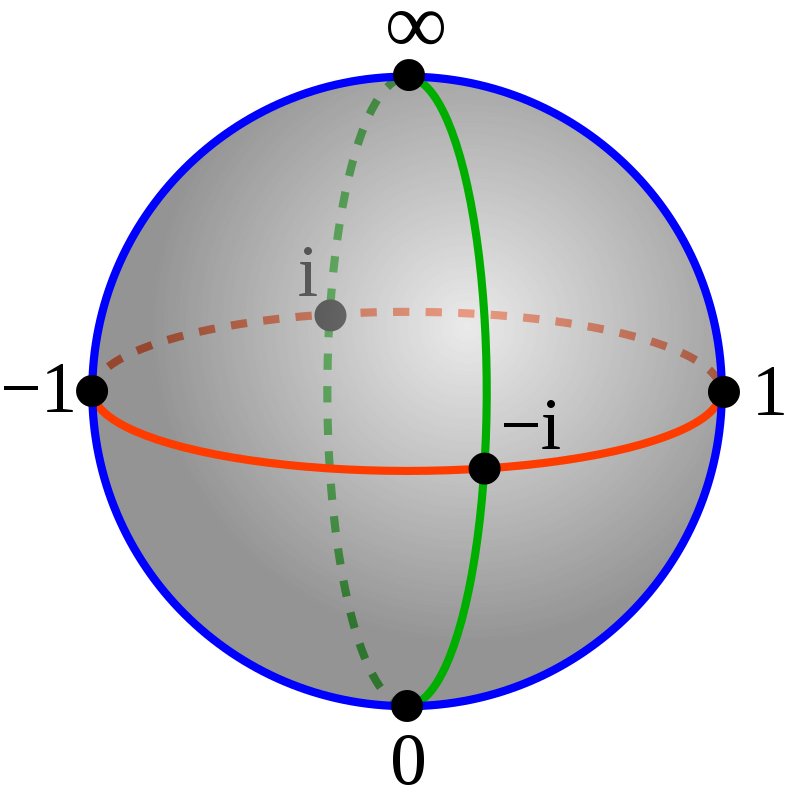
In the first illustration of the complex plane, relying on the XY axis, i appears at the top. In the second illustration of the Riemann Sphere, i is not at the top. Instead, i is on the equator, and infinity is at the top. I hadn't seen i from this equatorial angle before, so my mind started rotating the sphere to align with the coordinates in the first illustration. Intuition came alive, excited by what I was seeing. I felt a glimpse of something I'd seen before only in distant thought experiments, which I knew was remarkable. The glimpse was fleeting. I first tried rotating the sphere in my mind to capture the idea. Then, to think about it properly, I imported the image into Paint.NET and modified it to my liking. Aha! Yes, that's what I glimpsed! (See below). Great, but... working on this new image led to another insight, which then led to another, and another. I soon ended up with several modifications, each one marking another step in the journey my mind was making as I thought about the Riemann Sphere and the Complex Plane from another angle.
If you're already familiar with Imaginary Numbers, the Complex Plane, and the Riemann Sphere, you'll be able to follow along just by looking at the illustrations below. If not, start with the Wikipedia article, then follow along with the narrative as well: To begin, here is the original illustration from the article on Wikipedia:

I began rotating the image in my mind, to make it correspond better with previous thought experiments. Here is how I rotated it, putting infinity on the backside opposed by zero on the front (if you know how an aircraft moves, this 90-degree rotation is called "pitch"), and i, -i at top and bottom, like this:
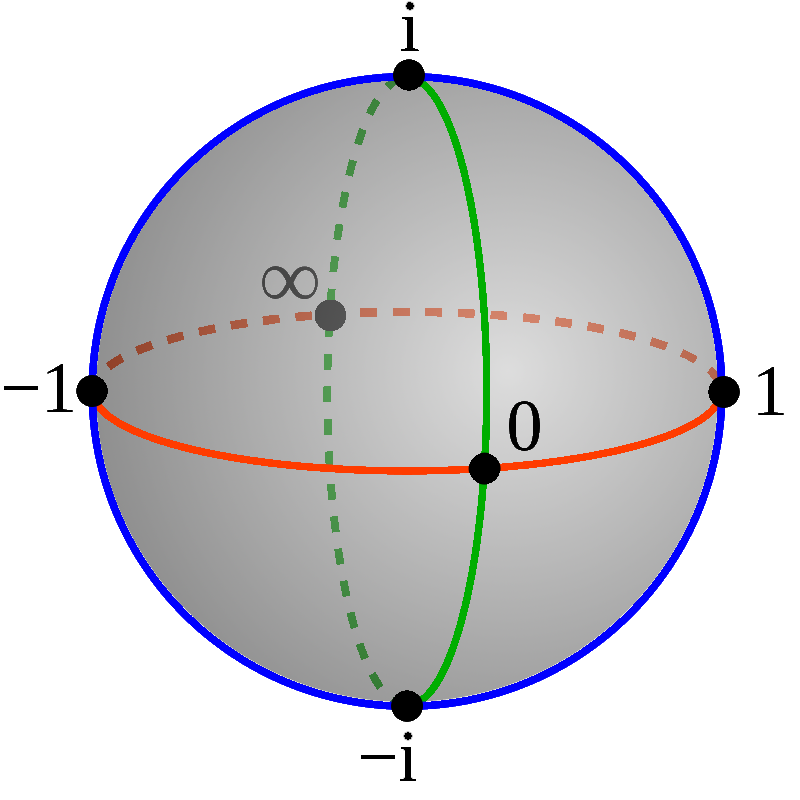
(If it seems somehow strange to see this, look at it closely and you'll see I simply rotated everything "top-backward" around the real axis which remained in place. This is a perfectly legal rotation which seems strange only because we do not normally see infinity and zero opposed along a lateral axis -- usually they're illustrated on a polar axis. But the -i and i in the polar regions should be familiar to some, as can be seen in the 2D image from Better Explained.
Once I finished that image, I realized something I had only speculated lightly before, although others have certainly known it: "1" should properly be known as "1r", especially when comparing it with "i", which itself should properly be known as "1i". (Only later did I discover that imaginary numbers cannot be ordered so this 1i assignment isn't necessarily in the same unit circle as 1r (?). As you can see, I believe that it is, but that's another topic altogether. The point here is, sometimes not knowing certain things can be a good thing.) I modified the illustration a little more, to make this clear:
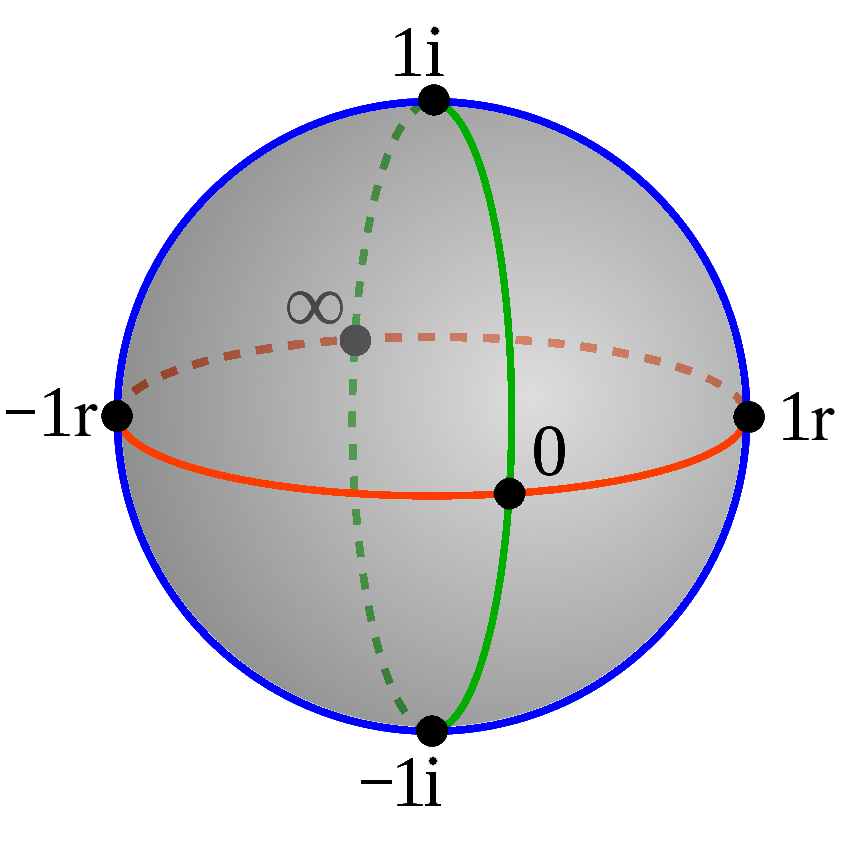
By now I had been working with the image for an hour and could clearly see that the new perception correlated very strongly with a whole other branch of previous thought experiments about how Infinity and Zero are the same thing. I've known and written about this for years, but never before was there such a concise illustration of the idea as I was now looking at. Seeing it in fragments and pieces over long stretches of time, I did not imagine it would be so easy to see this all at once, and there it was before me. Being able to see more of the picture, my imagination brought 0 and infinity together into the center, another move which I long contemplated, but had never really pursued before simultaneously. (Like everyone else, I had always accepted the common description with infinity being the North Pole -- an image which works really well with projective geometry. Even if I had tentatively moved it from the North Pole to the Center, I never suspected that I should also move the South Pole inward simultaneously, partly because until this present insight I hadn't realized how zero and infinity are sometimes drawn as opposites.). To capture this new idea, still just a hunch, I added the question mark you see below.
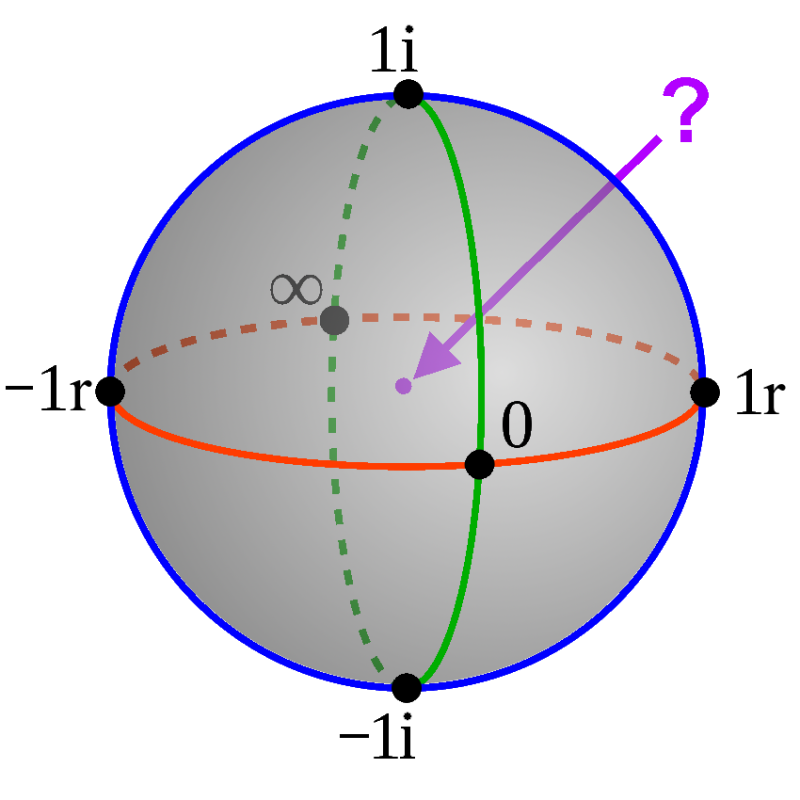
At this point, more fragments which were circulating around for years started to come together, and I soon wrote the small article which appears in a preceding blog post. Writing that post kind of put this current narrative on hold for a few days. If you read that little article you'll see where I'm going with this, or you can just continue stepping through the illustrations here... Oh good heavens. Just now, while writing (7:38a Mar 19), I was looking at that preceding image, thinking to myself "If zero and infinity are in the center, then what do we label the dots, to which they are pointing, in this image?" and the answer was something else I've contemplated for years. It's the same meditation Hamilton studied for years and years and years until one day, while walking across a bridge, he realized the formula which started Quaternion math: i² = j² = k² = ijk = -1. I understood his journey, for I contemplated along the same lines for years before I finally understood that Hamilton had already done the work. Like him, I had been searching for a triplet. Realizing that it requires a quadruplet to complete things was not obvious... until I finally understood that Quaternions are the solution to the "Triternion" puzzle. I'm going to modify the image again so you can see what I mean:
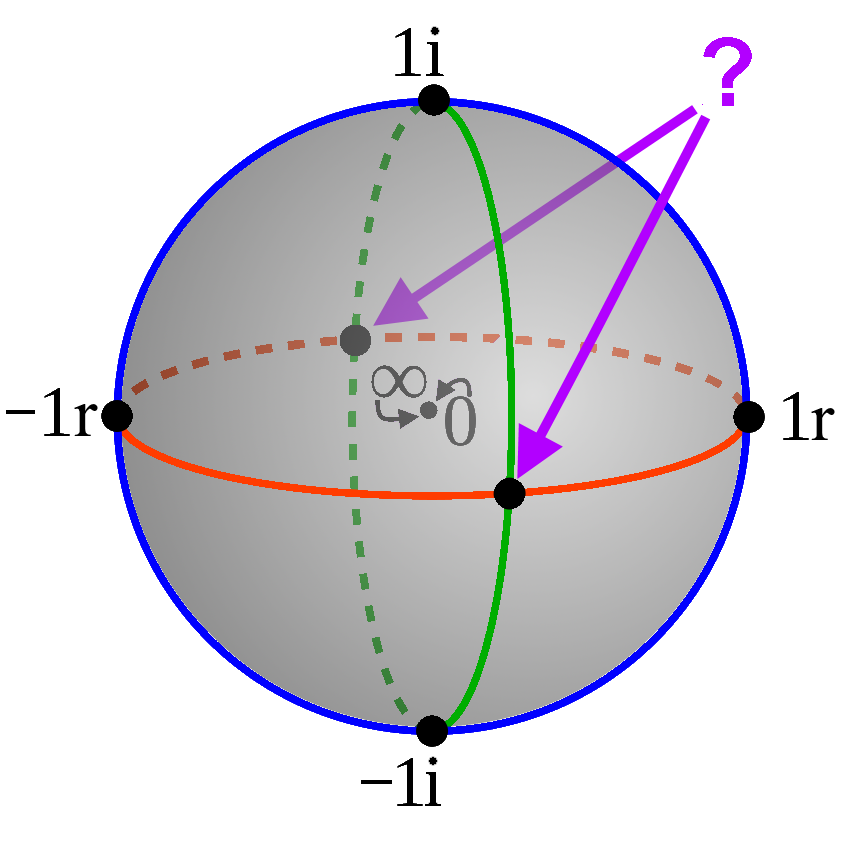
(Minutes later.) Well that didn't take long. As soon as I asked the question (in the previous paragraph) of my intuition, I could see the answer. I think you'll see it quickly, too. I'm keeping the 1r nomenclature instead of using 1k because there are two worlds merging, and continuing to use 1r (instead of 1k) makes the merge explicit.
Wow. I see it now. This is it! This explains why quaternion math is so useful when manipulating 3D space, such as in video game rendering and other similar applications, like the wave equation in quantum physics. It really is just manipulating 3D real space! Who ever knew reals have a spherical 3D dimension that aligns perfectly with imaginaries? Imaginary is not "imaginary" or even "lateral" (as Gauss wanted to call it), it is simply another dimension of reals. I was nearly done modifying the image one more time before I could see it, but you'll see it immediately if you know what you're looking for: Quaternion i, j, and k are also referencing the X, Y, and Z dimensions of the Real universe. (We never talk about the Y and Z dimensions of the Real universe, because we always imagine it as being a single linear universe.) In the R universe, the Complex Quaternion "k" coincides with the same point that I've been calling "r" in my images up to now. Look at this:
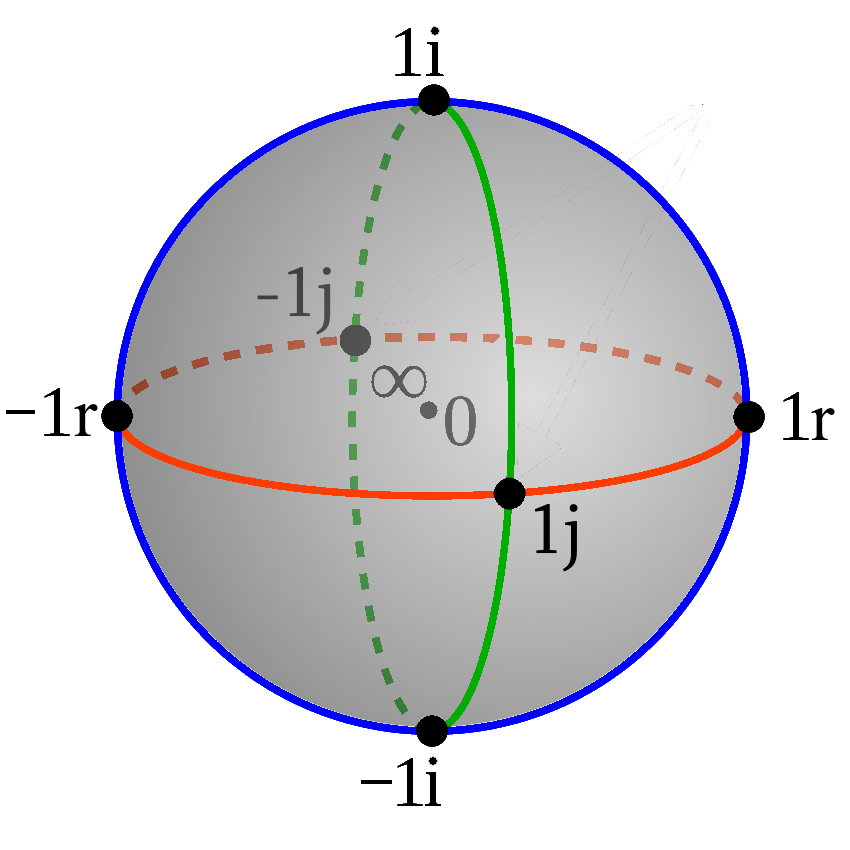
Finally, to make the point conveyed by this image complete, I merged the symbols for Zero and Infinity together and ended up with something that looked, um, zerofinity? It was coherent but it looked more complicated than a symbol for such an essential simplification should look. We're talking about a point that represents the center of everything, so we need something simple to convey this central point.
My attempts at nomenclature revealed the complexity: I first called this new symbol "zero plus infinity," then, "infinity with three bumps," then, for a while "trifinity" seemed like an elegant name for this zerofinity idea, as the "tri-" prefix hinted at hidden insights from ternary logic which I studied for years in order to come up with the various fragments which led to this thought experiment. But as I thought about it more, I realized it won't work: sadly, "trifinity" loses the "in" aspect of "infinite," making it into a reference to "three finite things" instead of "going beyond finite" or "without end." Trying to include "in" with something like "trinfinity" is... awkward, like the name of a new tech device used to help trim weight, which will only be on the market for a few months before the startup collapses because it has such a lame marketing name.
Eventually I reverted to calling this discovery either Spherical or Triternion Math and leaving the symbol out of things til something better evolved organically. [Update: and then today (April 19) happened. Long story short: the new symbol is called a synarché, which is coined from Greek "syn" and "arché". It is intended to be the intentionally-literal exact-opposite of infinity (or apeiron, both of which mean "without end.") Along the way of researching various new words, I discovered that the handsomest vortexy-squiggle character I ever met is the Greek letter "Xsi" or "Ks," spelled "?" in lowercase and "?" for uppercase. As a letter, its original meaning is "detached from" which is what the three dashes represent -- the middle bar is detached from what is above and below -- which is ironic because the full prefix "ksun" or "syn" (???) means "with". The full details of this side adventure are written in another place.]
synarché |
 |
| The Greek letter "?" pronounced "xsi" representing the first letter of the Greek word transliterated as "synarché" to name the combined point of zero and infinity. This includes both positive and negative infinities with zero at the center of a modified Riemann Sphere. This more accurately describes an independent "with-beginning" (syn-arché) rather than a dependent "without-end" (in-finity) which paradoxically describes something by reference to something that it is not. |
Now have a look at the completed illustration, where I've also shown how 1r is identical with 1k:
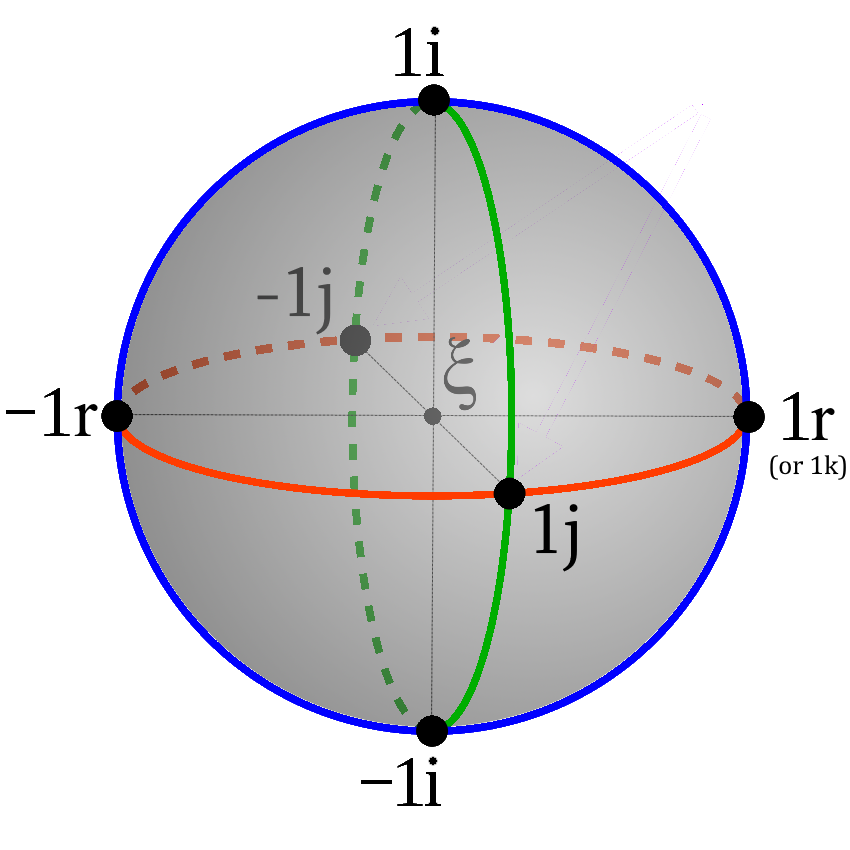
This is delightful. I've been pursuing this image by pure intuition from many directions for a dozen years or more. To see it here on the page in front of me is a stunning revelation, very pleasant to behold. There is a lot more going on here than this skeleton image shows, but it very elegantly reveals the core intuition upon which many other threads connect. (Hint for those who study Riemann: I suspect there's a whole adventure into the meaning of 1/2 in relation to this model which is yet to be explored.)
Please, if you got this far without reading this accompanying article, there is a lot of crossover between these two. Go read it so you can get the overall picture. The two (and the third one I linked previously) need to be combined into a single larger article which... if it all fits together as neatly as it seems now... should be published more formally. I have a feeling more people will want to see a proper treatment of these ideas... if they still cohere after a lot of inspection.
I know, I know, I need to study this out more and see how it works; there might be some technical issues with it, or it might also be entirely incoherent, but I think I've finally got it, exactly as I intuited in small pieces for years, now all together for the first time in one place.
[initial draft 8:45 a.m., Friday, March 19, 2021]
[edited for clarity, adding illustrations and links, published 10:45]
[note that the initial thought experiments started March 11]
[11:09 a.m. Triternions. That's what we'll call this space. Triternions are Quaternions merged with Reals by combining zero and infinity into trifinity synarché and aligning everything else from there. It's what Hamilton was seeking for so long, but didn't know that the compound interest from many centuries of the Law of Excluded Middle was preventing him from seeing how zero and infinity are the same thing and therefore i, j and k are simply the 3D Cartesian axes which align nicely with the Reals. It's another example of how the Law of Excluded Middle obscures and distorts intuition in strange, unexpected ways, as mentioned in the preceding article.]
[11:38, now thinking that the commutative complexity of Quaternions may be resolved by this approach, likely because it's in place to ensure there isn't a conflict with Reals. But that's just a hunch, based on my ideas that equality, addition, multiplication, division, etc. are none of them as simple as they look. Obviously, there is a lot buried in here that needs to be carefully investigated.]
[Updated a month later with the synarche section, April 19-20, 2021.]
